Quantum information

For the journal with this title, see Historical Social Research.

In quantum mechanics, quantum information is physical information that is held in the "state" of a quantum system. The most popular unit of quantum information is the qubit, a two-level quantum system. However, unlike classical digital states (which are discrete), a two-state quantum system can actually be in a superposition of the two states at any given time.

Quantum information differs from classical information in several respects, among which we note the following:

However, despite this, the amount of information that can be retrieved in a single qubit is equal to one bit. It is in the processing of information (quantum computation) that the differentiation occurs.

The ability to manipulate quantum information enables us to perform tasks that would be unachievable in a classical context, such as unconditionally secure transmission of information. Quantum information processing is the most general field that is concerned with quantum information. There are certain tasks which classical computers cannot perform "efficiently" (that is, in polynomial time) according to any known algorithm. However, a quantum computer can compute the answer to some of these problems in polynomial time; one well-known example of this is Shor's factoring algorithm. Other algorithms can speed up a task less dramatically—for example, Grover's search algorithm which gives a quadratic speed-up over the best possible classical algorithm.

Quantum information, and changes in quantum information, can be quantitatively measured by using an analogue of Shannon entropy, called the von Neumann entropy. Given a statistical ensemble of quantum mechanical systems with the density matrix \rho, it is given by

S(\rho) = -\operatorname{Tr}(\rho \ln \rho). \,

Many of the same entropy measures in classical information theory can also be generalized to the quantum case, such as Holevo entropy and the conditional quantum entropy.

Contents

Quantum information theory

The theory of quantum information is a result of the effort to generalise classical information theory to the quantum world. Quantum information theory aims to answer the following question:

What happens if information is stored in a state of a quantum system?

One of the strengths of classical information theory is that physical representation of information can be disregarded: There is no need for an 'ink-on-paper' information theory or a 'DVD information' theory. This is because it is always possible to efficiently transform information from one representation to another. However, this is not the case for quantum information: it is not possible, for example, to write down on paper the previously unknown information contained in the polarisation of a photon.

In general, quantum mechanics does not allow us to read out the state of a quantum system with arbitrary precision. The existence of Bell correlations between quantum systems cannot be converted into classical information. It is only possible to transform quantum information between quantum systems of sufficient information capacity. The information content of a message 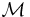\mathcal{M} can, for this reason, be measured in terms of the minimum number n of two-level systems which are needed to store the message: 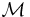\mathcal{M} consists of n qubits. In its original theoretical sense, the term qubit is thus a measure for the amount of information. A two-level quantum system can carry at most one qubit, in the same sense a classical binary digit can carry at most one classical bit.

As a consequence of the noisy-channel coding theorem, noise limits the information content of an analog information carrier to be finite. It is very difficult to protect the remaining finite information content of analog information carriers against noise. The example of classical analog information shows that quantum information processing schemes must necessarily be tolerant against noise, otherwise there would not be a chance for them to be useful. It was a big breakthrough for the theory of quantum information, when quantum error correction codes and fault-tolerant quantum computation schemes were discovered.

See also

Journals

Among the journals in this field are

External links and references

General
Quantum communication
Quantum algorithms
Quantum complexity theory
Quantum computing models
Decoherence prevention
Physical implementations
Quantum optics
Ultracold atoms
Spin-based
Superconducting quantum computing